Instantaneous Waterhammer Equation
With some exceptions, it is safe to calculate the maximum possible transient fluid pressure surge by using the instantaneous waterhammer equation.
Note: The instantaneous waterhammer equation is often credited to Joukowsky and referred to as the Joukowsky Equation.
Derived from the mass and momentum equations, this equation calculates the pressure change (ΔP) when instantly changing the flow velocity (ΔV) for a given density (ρ) and wavespeed (a):
There are cases where this value can be exceeded - these are discussed below in the Exceptions section.
Example
Assume flow of water is instantaneously stopped by a valve closure.
ρ = 62 lbm/ft3
a = 4000 feet/sec
ΔV = 10 feet/sec
Psteady = 50 psig
Exceptions
-
Cavitation
When a positive pressure surge occurs, it is always followed by negative pressure surge that drops the pressure. If the drop in pressure causes cavitation, then the pressure spike that occurs when the cavity collapses can cause the local pressure to exceed that predicted by the instantaneous waterhammer equation.
The potential to cavitate can be assessed by subtracting ΔP from the steady-state pressure. If this value is lower than the fluid vapor pressure, then cavitation is possible.
-
Pipe Size Changes
When using this equation, the case considered is usually a valve closure. It is frequently applied with the ΔV obtained based on the pipe diameter at the valve. However, if smaller diameter pipes exist upstream that carry the same flowrate, their ΔV will be larger because they have less area. This will result in a larger pressure surge in the smaller pipe.
To be safe, the equation needs to be applied to the pipe with the largest ΔV potential in the entire system.
-
Multiple Pressure Surge Sources
If there is potential for multiple elements to generate pressure surge at the same time, the surge pressures can interact and add together, thus exceeding the individual surge pressures.
-
Line Pack
In long pipelines or shorter systems with high friction the drop in Hydraulic Grade Line may be higher than the initial pressure surge. In these cases, the flow does not come to a complete stop with the initial compression wave - some flow continues towards the closed valve at a lower velocity, compressing the fluid and therefore increasing the pressure. See Wylie (1993)Wylie, E.B., V.L. Streeter & L. Suo, Fluid Transients in Systems, Prentice Hall, Englewood Hills, New Jersey, 1993. for more information.
Derivation
Consider a 1 dimensional fluid flow where the local velocity has been disturbed a small amount (ΔV). This small disturbance causes small disturbances in pressure (ΔP) and density (Δρ), along with a wave that travels with speed a. This is represented in the below control volume.
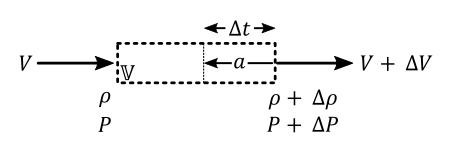
Figure 1: A downstream change in velocity causes a change in density and pressure, which propagates upstream as a wave.
First, we apply conservation of mass to the control volume above.
The left side of the equation, relating flow into and out of the control volume, reduces to:
The right side of the equation, relating the change in mass within the control volume, reduces to:
The density is only changing in the area swept by the wave front (moving at speed a, against the fluid velocity) over the time step. Therefore, we only need to include the change in density over this volume, as the other terms would cancel out. The volume swept by the wave is:
reducing the above to:
equating the sides and canceling the common A term:
Next, applying conservation of momentum:
The forces involved are simply the pressures over the areas. Because the pipe is considered frictionless for this method, the upstream and downstream pressures are equal.
The right hand side of the equation can be expanded into a time derivative and flux term:
Where the time derivative term follows similar logic to the mass conservation, with the volume affected defined by the swept area of the wave:
The flux term is relatively straightforward:
Combining and simplifying:
The two boxed formulas, representing conservation of mass and momentum, can be combined and simplified to the instantaneous waterhammer equation:
Related Topics



