Herschel-Bulkley Model

The Herschel-Bulkley model for non-Newtonian fluids is one of the most common viscosity models used in hydraulic analysis. This model states that the shear rate resulting from a given shear stress applied to the fluid can be described with the following piecewise equation:

Where du/dy is the shear rate (or velocity gradient), τ is the applied shear stress, τ_o is the yield stress, K is the consistency coefficient, and n is the flow behavior index.

The Herschel-Bulkley model describes describes η, the apparent viscosity of the fluid as:

In the Herschel-Bulkley model a value for τ_o of 0 and a value for n of 1 would result in a Newtonian fluid. The Herschel-Bulkley model is equivalent to the Bingham Plastic model if n = 1, and is equivalent to the Power Law model if τ_o = 0.

To employ this model, users must have values for τ_o, K and n, or have rheological data.

Herschel-Bulkley Interface

The Viscosity Model panel appears as shown below in Figure 1 when the Herschel-Bulkley model is selected.

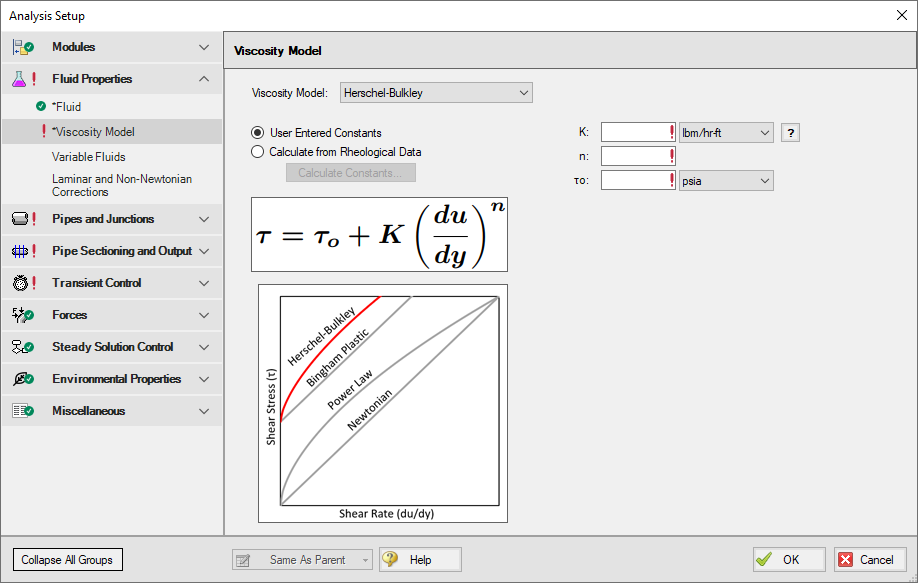
Figure 1: Viscosity Model panel for the Herschel-Bulkley model

Users must enter values for the Herschel-Bulkley constants, K, n, and τ_o. If the user has raw rheological test data instead, they can enter that directly using the Calculate from Rheological Data option.

Theory and Correlations

AFT Fathom models the frictional losses due to a Herschel-Bulkley fluid using correlations as are summarized in Garcia & SteffeGarcia, E. and Steffe, J., "Comparison of Friction Factor Equations for Non-Newtonian Fluids in Pipe Flow," Journal of Food Process Engineering, Vol. 9, pp. 93-120, April 1986. [Online]. Available: https://doi.org/10.1111/j.1745-4530.1986.tb00120.x (1986). Note that the original equations in the reference use the Fanning friction factor, while AFT software uses the Moody friction factor.

The laminar flow friction factor is defined as

with

and ξ_o, the dimensionless unsheared plug radius:

The turbulent flow friction factor can be calculated as follows

where

Where He is the Hedstrom number for Herschel-Bulkley fluids and is defined as

Re is the Reynolds number for Herschel-Bulkley fluids, and is equivalent to the Reynolds number for Power Law fluids:

The critical Reynolds number, which represents the transition from laminar to turbulent flow for Herschel-Bulkley fluids is defined as

Where n is the flow behavior index, Ψ_c is equivalent to Ψ with ξ_o = ξ_oc, and ξ_oc is defined implicitly as follows

The friction factor calculated using this correlation is then used to determine pressure loss following in the Network Solution Methodology topic.

These correlations are valid across the full range of Reynolds number. The applicability of this method to highly turbulent flow is limited only by the test data used to determine the Herschel-Bulkley constants.